Pressure Broadening
Eli has been a happy hare with the new chewy carrot colored Spectral Calculator toy the mice left in the burrow. He is digging out the effects of increasing CO2 concentrations on the greenhouse effect from the spectroscopic point of view. Recent posts on Real Climate by Spencer Weart and Ray Pierrehumbert touched this off, and, of course Motl's folly aggravated the lab bunnies beyond the breaking point. Yesterday Eli looked at the effect of temperature on the CO2 bending mode absorption spectrum. Today we are under pressure. If you don't know much about pressure, you could do a very lot worse than looking at our mob blogging friend Tamino's Introduction to the Gas Laws and his post on Pressure and Height, how pressure varies with altitude.
P(z) = Po exp (-g u z/ kT)
where z is the altitude, g the
We will use the Spectral Calculator to look at this. First, let us look at the 300 K CO2 absorption spectrum (mice wanting to play along should fire up Spectral Calculator and follow the instruction in the preceding post on Temperature
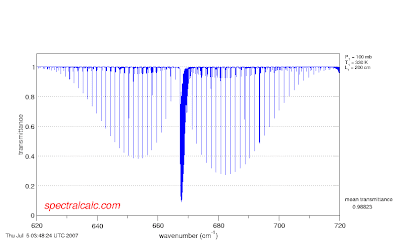We want to select a couple of lines from this, so after setting all the parameters as before (CO2 gas, the O(16)C(12)O(16) isotopomer, 10 cm cell length, 296K, 100 mbar total pressure, 0.000380 volume mixing ratio CO2) go to the observer page and set the upper and lower wavelength ranges as 680 and 684 cm-1. You will then see this spectrum
If we now increase the total pressure to 1000 mbar (~one atm), keeping the mixing ratio constant the result is
and looking carefully (you might have to click on each graph to bring up full screen images) we see that the transmission at each of the peaks in both spectra is ~93% (7% of the incident light is absorbed).Wait a minute. The volume mixing ratio in both spectra is 380 ppm. The total pressure in the first case was 100 mbar. The total pressure in the second case was 1000 mbar, ten times greater. That means that the amount of CO2 is ten times greater. We can, of course, keep the amount of CO2 constant by decreasing the mixing ratio in the second case to 38 ppm.
Transmission at the line centers is not 99.3%!. If we carefully integrated under each peak in this spectrum and the one taken at 100 mbar the integrated amount of absorbed light would be the same, just that the peaks are wider and shorter at 1000 mbar. Total absorption does not increase due to pressure broadening (within limits, see below), but it is spread to a wider range of frequencies /wavelengths.Finally a bit of ear waving about the origin of the pressure broadening. An isolated molecule's energy levels are shifted slightly when another atom or molecule nears and their electric fields interact. This changes the wavelengths at which molecules can absorb or emit. At low pressures only a relatively few molecules will collide at any instant, and pressure broadening occurs in the so called binary collision limit.
One can order the strength of electric interactions as ion > dipole > neutral. As a general rule, broadening by an ion is much longer range and stronger than broadening by a neutral. Broadening by a molecule with a permanent dipole moment stronger than broadening by one without. O2, because it has a magnetic moment in the ground state (two unpaired electrons) will be more effective as a broadener than nitrogen.
Having simplified all this, Eli will now point to the brier patch where there are lots and lots of nettles to sting you. First, as the molecules pass near to each other, the interaction will mix nearby quantum states. Transitions can borrow strength from each other and the absorption coefficients in each line can change. Second, collisions are of finite duration and range, which has the effect of decreasing intensity in the wings and increasing that at line center. Third, if absorption is observed for a relatively long path length (km) and for a molecule with a relatively high mixing ratio (CO2, H2O) absorption due to broadening can be significant 30 cm-1 or more away from the line center.
Now THAT would be a post.
UPDATE: If you want to learn about temperature effects see here and for more on pressure broadening see here and finally here
Posts
13 comments:
Minor quibble, about an error that one sees perpetuated more often than it should be: g is not the gravitational constant (which is G ~ 6.674 × 10^-11 m^3 kg^-1 s^-2 and is substantially constant) ... g is the acceleration due to gravity (which is ~9.81 m s^-2 and not particularly constant).
Keep up the good work guys (and dolls and bunnies).
Eli - At low pressures only a relatively few molecules will collide at any instant, and pressure broadening occurs in the so called binary collision limit.
On average, zero, according to my math. But it's not the number colliding at an instant that's important, its the number colliding during the emission time.
You say that total absorbtion cross section is not affected by pressure broadening. Why is that the case? I would (probably naively) expect that pressure broadening would increase total absorbtion.
Anon 4:29, we are horribly embarrassed and contrite.
CIP the broadening (to first order) simply spreads the absorption cross-section. Think of if as stomping on a Lorentzian (natural line shape till it forms a flat Gaussian. A similar question (which we can look at later, is does Doppler broadening increase total absorption.
It's very surprising to to me that the %transmittance at the line center would be the same (93%) on your first and second graph, even though the amount of CO2 differs by a factor of ten.
I understand that it's the total area under the line that determines total absorption, but it just seems surprising to me that all the additional absorption is being done off center.
I would have expected the absorption on center to change a little bit, at least. Perhaps it did, but if so, not by much (not that I can make out on that graph, at any rate).
This has to be more than coincidence. What is the physical reason why the absorption on center does not change?
A truly persipicacious question anon. The quick and dirty answer is that both the number density and the half width of the broadening are proportional to the total pressure.
This means that the added absorption from more CO2 gets spread out in the same proportion by the broadening.
Only when second order effects become important will this change. I want to fool about a bit tonite and see if I can write a meaningful update on this point
Eli - Think of if as stomping on a Lorentzian (natural line shape till it forms a flat Gaussian.
Some of technical terms are hard for me to understand. Let me see if I can translate that into everyday language: the transition amplitude for each molecule is proportional to the square of the matrix element. The volume absorption coefficient comes from summing these over the number of final states, essentially one per molecule-line. When some of these states are collisionally or otherwise shifted from the ground state (squashed) the number of atoms with shifted states has to be subtracted from those at line center (stomping). If this is so, I understand why the integrated absorption coefficient stays constant.
For sufficiently long paths, though, transmission could be nearly zero for line center and for the 1/e point, while still letting a lot through in between. In that case, pressure broadening could decrease the total absorption by expanding the (near) zero transmission region despite no change in the integrated absorption coefficent.
decrease the total transmission, I meant to say.
Here's my attempt at an explanation.
In the case where the pressure is increased but the amount of CO2 is kept constant (VMR reduced), the pressure broadening does not affect the total (integrated) absorption because:
1) the total number of molecules of CO2 available to absorb remains constant
2) The probability per unit time that any one of them will absorb a photon does not change
3) the number of photons available to be absorbed per unit time remains the same*
4) only the distribution of final energy states changes (which determines the wavelength of the photon that each molecule can absorb.)
Essentially, what has happened is that some of the molecules that, at low pressure, would have absorbed a photon with wavelength on line center now absorb a photon with wavelength off line center (either longer or shorter wavelength).
So, at higher pressure, fewer photons will now be absorbed that have wavelength on line center and the decrease is balanced with an increase in the total number of "off center" (higher and lower energy) photons absorbed.
*This assumes the number of photons available (per unit time) for absorption off line center is essentially the same as on line center, which will be (almost) true near the peak of the black body emission curve, where the emission intensity varies very slowly with wavelength.
Of course, this would not be true if the emission were concentrated within a narrow line (laser emission, for example). In that case, pressure broadening might have a significant effect on total absorption if the absorption line center is essentially coincident with the emission line.
--Horatio Algeranon
Horatio,
Nice explanation! Thanks!
A rather longer exposition on squashed Lorentzians and Gaussians
Hey, you got something against Lorentzians and Gaussians?
I count them among my best friends (right up there with the Lilliputians) and all this talk of "squashing them" has some of us mice a bit miffed.
Speaking of miffed, my spectral Calculator stopped working. It appears that they are now requiring me to subscribe.
There may be some limit on the number of accesses. I have not hit that yet.
Could we see some experimental evidence? Measured from real 10 cm length gas boxes? And see how well those results match up with the spectralcalc.com stuff...
That'd close up the argument pretty well.
-flavius collium
Post a Comment