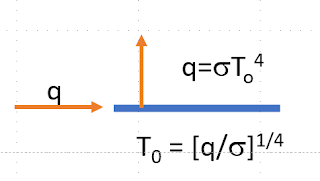To be honest, and Eli is always honest, good intentions slip away when one is sleeping through a lockdown, one day like another, and another and another and one simply stays in bed and does not visit Rabett Run muchly
However to continue where we left off, Eli has come across a a rather nice way of showing how absorption of thermal radiation in the atmosphere warms the surface.
Start with the simplest case the surface of an Earth whose atmosphere does not absorb infrared radiation. Solar energy q falling on the surface warms it until the surface can radiate an equal amount of energy. The temperature at which this happens is given by the Stefan-Boltzman equation.
Ok, this is a bit simplified, no albedo, all the energy is absorbed and none reflected and the emissivity of the surface is unity, no view factor, but that can be added back in later, here all Eli wants to do is establish the principle.
What would happen if we added two absorbing layers between the surface and space
Since the heat, the net thermal energy passing between each layer has to be the same as the heat going into the surface, q, there are three equations. At the top, the heat, being radiated to space is just the same as the heat injected at the bottom, and is given by σT24.
Between the first and second layer the same amount of heat, q, is transferred. In this case, q is the net difference between the thermal energy transferred upwards as shown by the blue arrow and the backradiation from the top layer shown by the orange arrow. Note that at the top the backradiation equals the radiation emitted to space which in turn is equal to the radiation absorbed at the bottom. Conservation of energy and all that.
At the bottom, the heat transferred between the surface and the first layer is also q, but in this case the difference is between the upwards thermal radiation from the surface, σT04 and the backradiation from the first layer σT14
Three equation, add them up, and you get that 3q = σT04, solve for T0 and compare to the result with only a surface. The temperature of the surface is warmer by a factor of 31/4 or if you want numbers about 1.3.
By inspection (instructor speak for do the work) if you had N layers the surface would warm by a factor of N1/4 while the input and output of heat from the system remains constant.
Vacuum ovens use this principle with multiple heat shields to slow the transfer of heat from the inside to the outside and that is where Eli first came across them when he was but a little guy building his own systems. (not nearly as neat as this one). Looking closely there are about 7 layers to the cake here.
There are a lot of discussions on line of
one layer energy balance models for the Earth's atmosphere which explicitly include emissivity and albedo, coming up with an effective emissivity of about 0,77 across the entire IR spectrum, but the multilayer POV puts paid to the argument that backradiation can't make the surface warmer